3 Boxes Problem

[Napalmbrain]

Would that not create another possibility though?

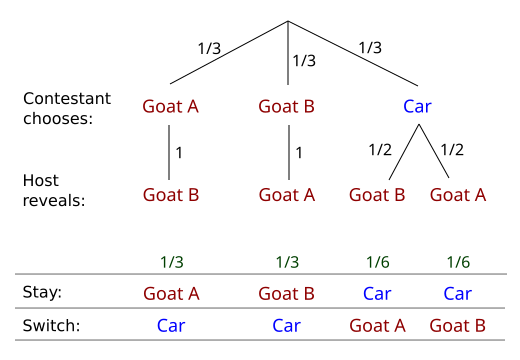
Not really. Basically, there are three choices: you can either pick the "good" box, or one of the "bad" boxes. If you picked the one of 2 "bad" boxes, then switch, you win. But if you picked the 1 "good" box, then switch, you lose. So assuming you switch, 2 possibilites result in a win, and 1 possibility results in a loss.

 

[Lewi T]

Excellent. I was hoping to be completely proved wrong, because I knew 2/3 was correct but I'd been looking for proof that switching is best, but everywhere I had looked people couldn't completely validate their answer.

 

[Sterculius]

It's all the same to me, because while the chances of success may theoretically change, the item within the box never changes boxes from start to finish. So while your chances get better or worse it's still all down to chance, no matter how many you're choosing from. Unless you're psychic.

 

[ROB64]

Bloody hell, never thought of it like that. Interesting...
I guess I should have taken statistics in my fifth year of maths instead of stopping at four years. :lol:

 

[LevesqueIsKing]

Oh, I get it. Because the box he removes can never be the box that you originally chose, so the yet-to-be-chosen box has 2/3 chance of being correct?

That took me forever to comprehend, and part of me still wants to believe that it's simply 50/50, but it's okay because I have yet to take statistics.

EDIT: That was a really interesting explanation, Napalm. That you want to originally pick a bad box so that you can switch to the good.

 

[gidget]

but what if you would have originally choosen an empty box?
the game host prolly wouldn't have opened an empty box to pressure you to change to the winning box...

 

[ROB64]

EDIT: That was a really interesting explanation, Napalm. That you want to originally pick a bad box so that you can switch to the good.

Yep, it was. That's what explained it for me, there's a 66.6666666% chance that you would pick an empty box, so you're chances are better if you switch, because it's more likely that you chose a box with nothing in it.

 

[Napalmbrain]

but what if you would have originally choosen an empty box?
the game host prolly wouldn't have opened an empty box to pressure you to change to the winning box...

The problem is based on the fact that the host always opens an empty box. It wouldn't work if he didn't.

 

[Chewy]

Took me a little while to get this but it actually makes a lot of sense if you think about it...

You're basically betting on the fact that you guessed wrong in the first place, which is a 2/3 chance.

 

[Foxy]

You are playing a game. A person (game host) shows you 3 boxes. 1 box contains £100, 2 others are empty. You need to choose one of the boxes. If the box you chosen contains the money you win.

Suppose you make a guess and point at some box. The game host instead of opening the box you just pointed at opens one of the other boxes and shows you that that box is empty. Then he asks "I'm going to give you a last chance to change your decision". So you can either stand by your original choice and point at the same box or choose the other one. What gives you better chances? (i.e. what are the chances you win if you choose the box you originally selected vs. the other box?)

Bloody Monty Hall, my maths teaches freaking loves it. Gets so giddy when talking about it.

 

[Barris1024]

wow this thread is amazing. The dude who created this, you are now my hero :lol:
That's so cool how it actually works. Now i need to remember this in case i'm ever on the price is right.
great thread, very interesting

 

[Lewi T]

wow this thread is amazing. The dude who created this, you are now my hero :lol:
That's so cool how it actually works. Now i need to remember this in case i'm ever on the price is right.
great thread, very interesting

 

[ProShooter]

Haha, morons.

edit: Think of it as two sides. There's your side, and the host side. When you choose one, the chance of having the hundred pounds in your side is 33% and his side is 66%. When he opens one of his and shows that it's empty and asks if you want to switch, you say yes, because his side still has a 66% chance of being the good box.

Edit: In case the first line seemed too offensive, know that I thought this at first as well.

:wtf: